- Overview
- Package
- Class
- Use
- Tree
- Deprecated
- Index

See: Description
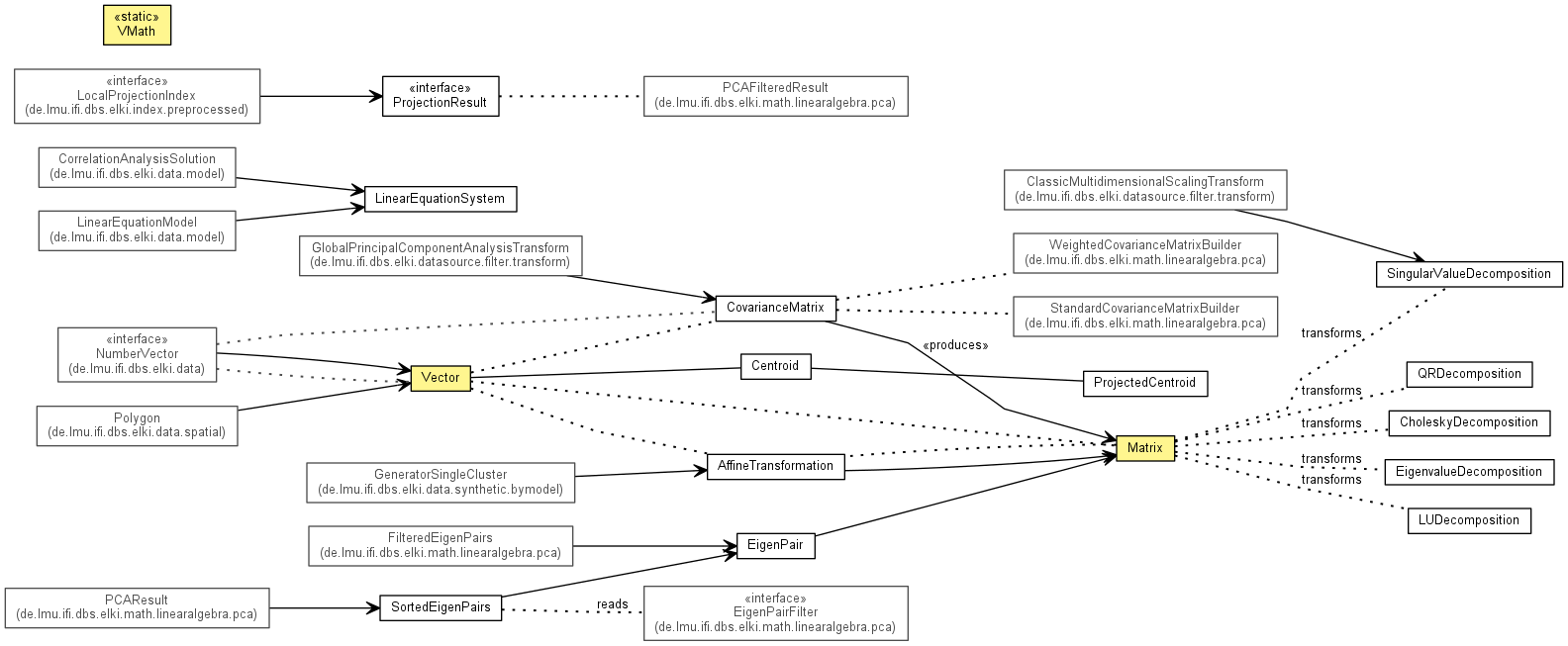
| Interface | Description |
|---|---|
| ProjectionResult |
Interface representing a simple projection result.
|
| Class | Description |
|---|---|
| AffineTransformation |
Affine transformations implemented using homogeneous coordinates.
|
| Centroid |
Class to compute the centroid of some data.
|
| CholeskyDecomposition |
Cholesky Decomposition.
|
| CovarianceMatrix |
Class for computing covariance matrixes using stable mean and variance
computations.
|
| EigenPair |
Helper class which encapsulates an eigenvector and its corresponding
eigenvalue.
|
| EigenvalueDecomposition |
Eigenvalues and eigenvectors of a real matrix.
|
| LinearEquationSystem |
Class for systems of linear equations.
|
| LUDecomposition |
LU Decomposition.
|
| Matrix |
A two-dimensional matrix class, where the data is stored as two-dimensional
array.
|
| ProjectedCentroid |
Centroid only using a subset of dimensions.
|
| QRDecomposition |
QR Decomposition.
|
| SingularValueDecomposition |
Singular Value Decomposition.
|
| SortedEigenPairs |
Helper class which encapsulates an array of eigenpairs (i.e. an array of
eigenvectors and their corresponding eigenvalues).
|
| Vector |
A mathematical vector object, along with mathematical operations.
|
| Vector.Factory |
Vector factory for Vectors.
|
| Vector.ShortSerializer |
Serialization class for dense double vectors with up to
Short.MAX_VALUE dimensions, by using a short for storing the
dimensionality. |
| Vector.SmallSerializer |
Serialization class for dense double vectors with up to 127 dimensions, by
using a byte for storing the dimensionality.
|
| Vector.VariableSerializer |
Serialization class for variable dimensionality by using VarInt encoding.
|
| VMath |
Class providing basic vector mathematics, for low-level vectors stored as
double[]. |
Linear Algebra package provides classes and computational methods for operations on matrices.
The content of this package is adapted from the Jama package.
Five fundamental matrix decompositions, which consist of pairs or triples of matrices, permutation vectors, and the like, produce results in five decomposition classes. These decompositions are accessed by the Matrix class to compute solutions of simultaneous linear equations, determinants, inverses and other matrix functions. The five decompositions are:
double[][] vals = {{1.,2.,3},{4.,5.,6.},{7.,8.,10.}};
Matrix A = new Matrix(vals);
Matrix b = Matrix.random(3,1);
Matrix x = A.solve(b);
Matrix r = A.times(x).minus(b);
double rnorm = r.normInf();
The original Jama-package has been developed by the MathWorks and NIST and can be found at http://math.nist.gov/javanumerics/jama/. Here, for the adaption some classes and methods convenient for data mining applications within ELKI were added. Furthermore some erroneous comments were corrected and the coding-style was subtly changed to a more Java-typical style.
Copyright © 2015 ELKI Development Team, Lehr- und Forschungseinheit für Datenbanksysteme, Ludwig-Maximilians-Universität München. License information.